Saxophone acoustics: an introduction

This diagram sketches what happens if you increase the pressure without changing the reed aperture. (Warning: what happens in playing more loudly is usually more complicated than this, because higher blowing pressure tends to close the aperture, which tends to reduce the flow, and splayers simultaneously reduce the bite force which—see the previous graph—increases the flow, all else equal.) Higher pressure moves the operating point to the right, and it also increases the range of pressure. This means that the (larger) section of the curve we use is no longer approximately linear. This produces an asymmetric oscillation. It is no longer a sine wave, so its spectrum has more higher harmonics. (Centre diagram.) Have a look at the spectra for different dynamic levels on the note A#3. Observe that the increase in the level of higher harmonics is much greater than that in the fundamental.

When we blow hard enough (and even if we relax the bite), the reed will close for part of the part of the cycle when the pressure in the mouthpiece is low due to the standing wave inside the instrument. So the flow is zero for part of the cycle. The resultant waveform is 'clipped' on one side (diagram at right), and contains even more high harmonics. As well as making the timbre brighter, add more harmonics makes the sound louder as well, because the higher harmonics fall in the frequency range where our hearing is most sensitive (See What is a decibel? for details).

While talking about decibels, we should mention that spectra, including those on our saxophone site are usually shown on a decibel scale. This means that one notices easily on the spectrum a harmonic that is say 20 dB weaker than the fundamental, even though it has 10 times less pressure and 100 times less power. What is important is that your ear notices it too, because of the frequency dependence referred to above. However, it is much more difficult to notice the presence of harmonics if you look at the waveform.

Soon after this page went up, saxophonists wanted to know a lot more about playing more loudly. The variation in pressure and flow (rather than the average values) are what make the sound loud: the length of the thick line in the sketched graphs above. Blowing harder makes the gain of the reed higher and that increases the amplitude of the standing waves in the bore. Further, blowing harder eventually takes you into the non-linear and clipping ranges that produce stronger high harmonics, and therefore a sound that is both louder and brighter. However, if you blow too hard, you close the reed completely and it stays closed. In practice, players relax the bite when they play loudly, so the whole curve moves up (from the lower red to the upper blue curve in the preceding graph).

You can prevent the reed closing with a harder reed. A harder reed requires greater pressure differences to bend, and so allows you to blow harder without entering the non-linear or clipping ranges. On the other hand, the smaller nonlinearity makes the sound more mellow (and therefore less loud, all else equal). This is complicated by the embouchure: because of the curvature of the lay, the position where you place your lower lip and how hard you bite makes a difference to the effective length and therefore stiffness of the reed. It's like a lever whose fulcrum is moved. Mouthpiece design also influences the amplitude at which non-linear or clipping ranges begin: a mouthpiece with an obstruction near the reed tip becomes less linear at lower pressures and so produces the brighter (harsher?) and louder sound beloved of some saxophonists. Alright, I admit it, I have such a mouthpiece in the case, too.

Many examples of sound spectra are given on the data base. To compare spectra at different loudness, go here.

Control parametes for playing

The saxophone is a 'closed' pipe

For the purposes of this simple introduction to saxophone acoustics, we shall now make some serious approximations. First, we shall pretend that it is a simple conical pipe – in other words we shall assume that all holes are closed (down to a certain point, at least), that the bore is conical, and that the mouthpiece end is completely closed. This is a crude approximation, but it preserves much of the essential physics, and it is easier to discuss. Of course the saxophone doesn't come to a sharp point: it has a mouthpiece. The mouthpiece is shorter and fatter than the cone it replaces, and it has approximately the same volume.

    

At left is a schematic of a soprano saxophone, an idealised conical bore and a truncated cone. At right is a photo of a Yamaha soprano saxophone. The larger saxophones are bent to bring the keys within comfortable reach of the hands. The bends make only modest differences to the sound, so we shall picture straight saxophones in the diagrams here. This link shows modern Yamaha soprano and tenor saxes, with a metre rule. This link shows three experienced saxophones (sop, alto and tenor) from the author's collection.

The natural vibrations of the air in the saxophone, the ones that cause it to play notes, are due to standing waves. (If you need an introduction to this important concept, see standing waves.) What are the standing waves that are possible in such a tube?

To answer the question, we must take into account the fact that the saxophone is approximately conical. This means that sound waves 'spread out' as they travel down the bell. This means that the amplitude of the waves gets smaller as we go from mouthpiece to bell. The fact that the saxophone is open to the air at the far end means that the total pressure at that end of the pipe must be approximately atmospheric pressure. In other words, the acoustic pressure (the variation in pressure due to sound waves) is zero. The mouthpiece end, on the other hand, can have a maximum variation in pressure: it is an antinode in pressure. If we were dealing with a cylindrical pipe (such as a flute or clarinet), where the standing waves are sinusoidal, we would expect the maximum and the zero of a wave to be one quarter wavelength apart. But the variation in the amplitude of the wave due to the variation in cross sectional area complicates the story.

So we have devoted a whole page to comparing cylindrical and conical pipes and, if you want the details, you should read that page now. However, the result is this: the standing waves in a cone of length L have wavelengths of 2L, L, 2L/3, L/2, 2L/5... in other words 2L/n, where n is a whole number. The wave with wavelength 2L is called the fundamental, that with 2L/2 is called the second harmonic, and that with 2L/n the nth harmonic. The frequency equals the wave speed divided by the wavelength, so this longest wave corresponds to the lowest note on the instrument: Ab on a Bb saxophone, Db on an Eb saxophone. (See standard note names, and remember that saxophones are transposing instruments, so that the written low Bb3 is actually Ab2 for a tenor saxophone in Bb, Db3 for an alto in Eb, and Ab3 for a soprano in Bb. Hereafter we refer only to the written pitch.) You might want to measure the length of your instrument, take v = 345 m/s for sound in warm, moist air, and calculate the expected frequency. Do you get a better answer if you use the real length, or if you use the length of the cone made by extrapolating back to a point? Then check the answer in the note table. The acoustic response of wind instruments is often quantified using the acoustic impedance spectrum, which we discuss below. This specifies how hard it is to make air vibrate at a given frequency, or what acoustic pressure is produced by air vibration at a given frequency. Saxophones operate at peaks in the impedance. The graphs above right show the impedance spectra for low Bb on a soprano and a tenor. The instrument will play several of these peaks – called bugling. Harmonics So, with all of the holes closed, you can play the lowest note: (written) Bb3, with a wavelength somewhat more than twice the length of the instrument. With this fingering, however, you can also play other notes by overblowing – by changing your embouchure and changing the blowing pressure. The harmonic series on Bb3 is shown below.

How the reed and pipe work together

When the saxophone is playing, the reed is vibrating at one particular frequency. But, especially if the vibration is large, as it is when playing loudly, it generates harmonics (see What is a sound spectrum?). These set up, and are in turn reinforced by, standing waves. Consequently, the sound spectrum has strong components at harmonics of the fundamental being played. For notes in the low range of the instrument, there are usually resonances at the frequencies of some of the harmonics. These result in greater radiation of the sound at those frequencies. In the high range of the instrument, the higher resonances do not systematically fall near harmonics of the note being played. See the data base of saxophone acoustics for examples.

Register holes are discussed in more detail on the page about clarinet acoustics. See more about register holes on the clarinet page.

Spectrum of the saxophone

Opening tone holes

For the moment, we can say the an open tone hole is almost like a 'short circuit' to the outside air, so the first open tone hole acts approximately as though the saxophone were 'sawn off' near the location of the tone hole. This approximation is crude, and in practice the wave extends somewhat beyond the first open tone hole: an end effect.

(For the technically minded, we could continue the electrical analogy by saying that the air in the open tone hole has inertia and is therefore actually more like a low value inductance. The impedance of an inductor in electricity, or an inertance in acoustics, is proportional to frequency. So the tone hole behaves more like a short circuit at low frequencies than at high. This leads to the possibility of cross fingering, which we have studied in more detail in classical and baroque flutes.)

The frequency dependence of this end effect means that the higher note played with a particular fingering has a larger end effect than does the corresponding note in the lower register. If the saxophone really were a perfect cone with tone holes, then the registers would be out of tune: the intervals would be too narrow. This effect is removed primarily by the the shape and volume of the mouthpiece.

Register holes

A small hole can serve as a register hole. For instance, if you play E4 (call this frequency f_o) and then push the octave key, you are opening a hole part way down the (closed part of the) instrument. This hole disturbs the resonance that supports the fundamental of E4, but has little effect on the higher harmonics, so the saxophone 'jumps up' to E5 (2f_o).

Where to put it? The acoustically obvious place to put a register hole is at a pressure node of the upper note which is also a region of large pressure variation for the lower note. Opening the bore to atmospheric pressure at a pressure node makes no difference to that note. The trouble is that each note in the upper register has its pressure node at a different position. One can imagine a saxophone that had a separate register hole for each note, but that would be a lot of keys. In fact, only two register holes are used for the second register from D5 to F#6. This is of course a compromise: the register hole is never at the pressure node of the standing wave of the required upper note. This is not a big problem in practice. The register hole is small, so it is not really a 'short circuit', except at low frequencies. So it does not too much affect the higher frequency standing waves. (We explain how the mass of the air seals the hole at high frequencies below.) It does however disrupt the fundamental, and that is its purpose: to stop the instrument dropping down to its bottom register.

Just in case you haven't noticed on your own saxophone, the octave key is automated: one key opens one or other of two register holes, according to whether or not the third finger of the left hand is depressed. So the upper register hole (right at the top near the mouthpiece) opens for notes above G#5, whereas from D5 to G#5 the lower hole is used. This is an example of a mechanical logic gate, which is well worth examining closely. The octave keys on oboes are only partly automated and on bassoons---trust me, you don't want to know.

The octave key register holes are used from D5 to F#6. In higher registers, other register holes are used. (Some players use the key for F6 as a register hole. This hole is designed primarily as a tone hole, so it is bigger than it need or should be for an ideal register hole. Some players adjust the mechanism for the alternate F6 fingering so that it opens that hole only a little way. This improves its performance as a register hole, but compromises the intonation of the alternate high F fingering.)

Cross fingering

An open tone hole connects the bore to the air outside, whose acoustic pressure is approximately zero. But the connection is not a 'short circuit': the air in and near the tone hole has mass and requires a force to be moved. So the pressure inside the bore under a tone hole is not at zero acoustic pressure, and so the standing wave in the instrument extends a little way past the first open tone hole. (There's more about this effect under Cut-off frequencies.) Closing a downstream hole extends the standing wave even further and so increases the effective length of the instrument for that fingering, which makes the resonant frequencies lower and the pitch flatter.

The effect of cross fingerings is frequency dependent. The extent of the standing wave beyond an open hole increases with the frequency, especially for small holes, because it takes more force to move the air in the tone hole at high frequencies. This has the effect of making the effective length of the bore increase with increasing frequency. As a result, the resonances at higher frequencies tend to become flatter than strict harmonic ratios. Because the saxophone's tone holes are large, cross fingerings have relatively little effect on the pitch in the first two registers. Nevertheless, comparing the two fingerings discussed above, one sees that the first open tone hole in the simple fingering is both smaller and further down the bore than the first open tone hole in the cross fingering.

A further effect of the disturbed harmonic ratios of the maxima in impedance is that the harmonics that sound when a low note is played will not 'receive much help' from resonances in the instrument. (Technically, the bore does not provide feedback for the reed at that frequency, and nor does it provide impedance matching, so less of the high harmonics are present in the reed motion and they are also less efficiently radiated as sound. See Frequency response and acoustic impedance. To be technical, there is also less of the mode locking that occurs due to the non-linear vibration of the reed.) As a result, cross fingerings in general are less loud and have darker or more mellow timbre than do the notes on either side. You will also see that the impedance spectrum is more complicated for cross fingerings than for simple fingerings.

We have studied cross fingerings more extensively on flutes than on saxophones, by comparing baroque, classical and modern instruments. (There is of course no baroque saxophone.) See cross fingering on flutes or download a scientific paper about cross fingering.

Other effects of the reed

As well as controlling the flow of air, the reed has a passive role in saxophone acoustics. When the pressure inside the mouthpiece rises, the reed is pushed outwards. Conversely, suction draws the reed in towards the bore. Thus the reed increases and decreases the mouthpiece volume with high or low pressure. (Techncially, we say it is a mechanical compliance in parallel with the bore.) Indeed, it behaves a bit like an extra volume of air, which could also be compressed and expanded by changing pressure in the mouthpiece. It has the effect of lowering the frequency of each resonance a little. However, soft reeds move more than hard reeds, so soft reeds lower the frequency more than do hard reeds. Further, this effect is greater on high notes than on low, so soft reeds make intervals narrower and hard reeds make them wider. This is useful to know if you have intonation problems. (See also tuning.)

Of course, the mouthpiece itself also contains a volume of air (an acoustic compliance) and so it has an effect on the tuning of the resonances. The combined compliance of the mouthpiece and reed is approximately equivalent to the volume of the cone that would be required to extend the body of the saxophone to a point.

Cut-off frequencies

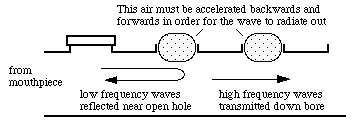
So high frequency waves are impeded by the air in the tone hole: it doesn't 'look so open' to them as it does to the waves of low frequency. Low frequency waves are reflected at the first open tone hole, higher frequency waves travel further (which can allow cross fingering) and sufficiently high frequency waves travel down the tube past the open holes. Thus an array of open tone holes acts as a high pass filter: some thing that lets high frequencies pass but rejects low frequencies. (See filter examples.) The cutoff frequency is about 800 Hz for the tenor and 1300 Hz for the soprano. In the sound spectra of low notes, you will notice that the harmonics fall off above this frequency. You will also notice that the impedance spectra become irregular above this frequency. See the data base of saxophone acoustics.

The cutoff frequency and the conical shape together limit the ability to play high notes on the saxophone, unless the player uses his/her vocal tract as a resonator instead. The stiffness of the reed is another: a saxophone will only play notes with frequencies lower than the natural frequency of the reed. (There is a more detailed explanation of cut-off frequencies and their effects here.)

Frequency response and acoustic impedance of the saxophone The way in which the reed opens and closes to control the air flow into the instrument depends upon the acoustic impedance at the position of the reed, which is why we measure this quantity. The acoustic impedance is simply the ratio of the sound pressure at the measurement point divided by the acoustic volume flow (which is just the area multiplied by the particle velocity). If the impedance is high, the pressure variation is large and so it can control the reed and the flow of air past it. In fact, the resonances, which are the frequencies for which the acoustic impedance is high, are so important that they 'control' the vibration of the reed, and the instrument will play only at a frequency close to a resonance. (There is further explanation on What is acoustic impedance and why is it important?). The section below shows how the major features of the saxophone's shape give rise to its acoustic impedance spectrum, and thus to how it operates. We repeat the graph of the impedance spectra of the lowest notes of soprano and tenor. We also have a data base of saxophone acoustics that shows saxophone impedances and sound files for many notes and some multiphonics. (We also have such a data base of clarinet acoustics. It's worth contrasting them: First, the soprano saxophone is actually a little longer than a clarinet – about 700 vs 670 mm. However, the first resonance of the clarinet (call it fo) occurs at a lower frequency than that of the saxophone (call it go). Further, the peaks of the saxophone curve occur at all the harmonics (go, 2go, 3go etc), whereas those of the clarinet curve occur only at the odd harmonics (fo, 3fo, 5 fo etc. The differences among open cylindrical pipes (flutes), closed cylindrical pipes (clarinets) and closed conical pipes (saxophones, oboes, bassoons) are explained in Pipes and harmonics. This difference gives the clarinets a big advantage: a clarinet of a given length can play lower notes than can conical instruments of the same length. (Baritone sax players envy the small case that bass clarinettists lug around.) Clarinets pay for this advantage: the odd harmonic series means that they overblow a twelfth instead of an octave, which makes the fingering more awkward, especially around the 'break' between registers. They are also less loud than a comparable conical instrument. (The baritone sax player can blow the bass clarinettist away on that score.) For frequencies above about several hundred hertz, the resonances become weaker with increasing frequency. This is due to the 'friction' of the moving air against the inside of the instrument (technically, the viscous and to a smaller extent thermal losses in the boundary layer). This affects higher frequencies more than low. However, a problem faced by the conical instruments but not by cylindrical instruments is that the first resonance is weak. We saw above under cut-off frequency that, at low frequencies, it is easier to move the air backwards and forwards, because less acceleration is involved. This means that the impedance of the 'air at the downstream end of the instrument' (technically the radiation impedance at the end) is low for low frequencies. Further, the cone is good at matching the low impedance of the large end to the high impedance at the small end. Together, these effects make the lowest resonance of conical instruments weaker than those of their cylindrical cousins. That is why the whole curve turns down to lower values at low frequency. (Compare with curves for the clarinet.)

Effect of the bell. The bell 'helps' the sound waves in the bore to radiate out into the air. (Incidentally, the presence of a large, effective bell is what makes brass instruments loud: try playing a trombone with the tuning slide taken off.) More sound radiated means less sound reflected, so the standing waves are weaker. However, this effect is only strong for high frequency: as the frequency increases over this range, the resonances are more weakened by the bell at high frequency than at low. This is because the bell is much smaller than the wavelengths of the low frequency waves, and so is less effective at radiating these waves. The bell cuts in at 1.8 kHz for the tenor and 2.6 kHz for the soprano. Above these frequencies the instruments have hardly any resonances – but they do behave as good megaphones!

The effect of the bell is that of a high pass filter. We could say that this is rather like the cut-off frequency effect of a series of open tone holes. In fact, one purpose of the saxophone's bell chiefly is to provide a high pass filter for the lowest few notes, so that they have a cut-off frequency and so behave more similarly to the notes produced with several tone holes open. As far as fundamental frequencies go, the bell is really only important to the lowest notes. However, it is important to the high harmonics of most notes.

Saxophone and oboe players will know the acoustic effect of this weak first resonance discussed above: it makes it difficult to play notes at the very bottom of the range. It is especially difficult to play these notes softly. We saw in How the reed and pipe work together that the higher harmonics of the reed motion could be 'helped' by resonances of the bore. When you play loudly, you generate proportionally more power in the higher harmonics (this is due to increasingly non-linear motion of the reed). So the lowest notes are not so difficult to play loudly, where the strong, high harmonics of the reed are supported by resonances of the bore, compared with soft notes, where you are relying on the the weak fundamental resonance.

We have not mentioned the effect of the mouthpiece. First, we note that the bore is not a simple cone: it is a cone truncated at a comfortable diameter to take a mouthpiece. This truncation affects the tuning: informally, we can think of it as making the pipe slightly like a cylinder, which stretches the frequency gap between resonances. This means that, unless compensated, it would stretch the interval between registers to over an octave. Now the geometry of the mouthpiece is a little complicated, but one contribution to the acoustic response is that it compensates for the 'missing volume' of the cone. Indeed, its volume (when added to the effective volume of the reed) is comparable with that of the missing cone. The compliance of the mouthpiece reduces the acoustic impedance spectrum over a range near about 1 kHz. See the data base for examples.

To the effect of the mouthpiece volume, we may add the compliance of the reed, discussed above. This acts in parallel with the bore, and its impedance decreases at high frequency, so its effect is to reduce the rise in impedance with frequency: softer reeds give lower overall impedance at high frequency. Further, the very high resonances are weaker and occur at lower frequency when you use a soft reed. This is discussed in more detail under the "effect of hardness" section on the clarinet page.

Vocal tract effects

More to come

We shall continue to add to this site as we find time and as our research results are published. One FAQ is 'Why don't we provide a Virtual Saxophone service like the Virtual Flute?' The answer is that we are working on the version for clarinet and hope to have one soon. The sax will probably be next.

Experimental measurements on this page were made by Jer Ming Chen as part of his doctoral research, and are described in the paper below.

Scientific papers with more detail:

• Chen, J.M., Smith, J. and Wolfe, J. (2011) "Saxophonists tune vocal tract resonances in advanced performance techniques" J. Acoust. Soc. America. 129, 415-426.
• Chen, J.M., Smith J. and Wolfe, J. (2009) "Saxophone acoustics: introducing a compendium of impedance and sound spectra" Acoustics Australia, 37, 18-23.
• Chen, J.M., Smith,. J. and Wolfe, J. (2008) "Experienced saxophonists learn to tune their vocal tracts", Science, 319, 726. A popular version is given in this link.