High school physics FAQ

Relativity

Inertial and non-inertial frames of reference.

Centripital forces and inertial frames

The thought experiment involving the mirrors on either side of a train is rather like Michelson and Morley's interferometer experiment, though the geometry is more complicated. In this case the Earth is the 'train' travelling towards and away from a distant star a 6 month intervals.

The relationship between thought and reality is the province of philosophers and, enjoyable though it be, I'd rather not trespass.

Mass defect

Mass dilation

Relativity and space travel

The short answer is "not very much". For space travel of the sort that we can conceive, the effects due to time dilation and length contraction are tiny. This does not mean that they cannot be measured: it is not highly difficult to measure the very small time dilation effect. But the practical implications are minimal.

Space travel

Please explain why the forces acting on an astronaut increase to approximately |3W| during the intial periods of launch.

What is the advantage of setting PE = 0 at r = infinity instead of having, lets say, the centre of the earth to be zero?

Gravitational PE at a distance r from the earth's centre is given by U = − GMm/r, where r > radius of the Earth, and U = 0 at r = infinity. The equation is only true when r is greater than or equal to the radius of the Earth. Newton wrote a nice theorem establishing this: for gravity, a hollow shell has no effect if you're inside it, and if you're outside it, all of its mass may be considered to act at the centre. So the equation involving the mass M of the whole earth only applies when you are outside the earth. So the centre of the Earth can't be used. Using the surface (r = rE) would be possible: this would give Urelative = − GMm/r + GMm/rE However, this is not used. It is more awkward, it has a new parameter to remember, and it is specific for one particular planet. The sketch compares the usual astronomical version (GMm/r, the solid line) and the local version (mgh, dashed line). mgh is a poor approximation for altitudes that are not negligible in comparison with the radius of the Earth.

The syllabus says 'Define gravitational potenial energy as the work done to move an object from a very large distance away to a point in a gravitational field.' Can anyone explain this? Why do we define gravitational potential energy?

1) We divide forces into two sorts: conservative forces (such as gravity) and nonconservative forces (such as friction). By definition, the work done during a round trip is zero for a conservative force. Example: I lift my pen from the desk, I do work against gravity. I lower it to the desk, gravity does work on me. Total work done in the round trip = 0. I slide the pen to the right, I do work against friction. I slide it back to the left and I still do work against friction. Total work done in the round trip > 0.

2) For an object acted on by a conservative force, we can define a potential energy due to that conservative force, as a function of position. The difference in potential energy (U_b - U_a) between points a and b is defined as the work done against that force to move the object from a to b. (You can now see why we can't do this for nonconservative forces: if we do a round trip from point b to point b, we do work against such forces and so the potential energy at b would have no unique definition.)

3) Gravity is a conservative force. So, for a body with mass m, we define the difference in gravitational potential energy between points a and b as the work as the work done against gravity to move mass m from a to b.

4) Note that the force may vary with position: the Earth's gravitational field gets stronger as we approach the surface of the Earth, from either direction. So we could say

dU = dW = -F.ds where F is the gravitational force, and so
U = - integral F.ds.
Notice that the integral introduces a constant of integration. This constant corresponds to a reference point for gravitational potential energy: in terms of the definition above, it is the gravitational potential energy at point a, where we chose a to be some convenient reference (see the section above).

"The main energy cost associated with space travel is currently fuel to reach Low Earth Orbit. Energy is also needed to leave this orbit, change direction/ accelerate and for communication. What else is there to say?"

It's true that we have to give a satellite the extra gravitational potential energy of its orbit, plus the kinetic energy associated with a speed that allows it to stay there.

But we actually give spend more energy than that, because we have to lift a lot of fuel. Most of the fuel doesn't go very high, but we still have to lift and to accelerate that fuel. So most of the energy goes into carrying fuel, and most of that fuel is there to carry more fuel, and most of that..... Which is why the Saturn V booster is a very big can of fuel.

If we fired the satellite out of a gun (as in HG Wells' "From the Earth to the Moon"), we would burn the fuel on the ground, and therefore not have to lift nor to accelerate it We would therefore need only a tiny fraction of the energy that is currently used. Unfortunately, the huge acceleration would damage the satellite and kill any passengers.

Microwaves have wavelengths measured in microns to cm. What we call radio waves are usually rather longer, say metres to many km.

In order to communicate over a long distance, you need to confine the radiated power to a beam of small cross sectional area, in other words to send out a nearly parallel beam. Then you need to intercept it and focus it. For both of these, you use parabolic dish, a little like those uesd for satellite television. Now the whole idea of rays and focussing only works well for dishes much bigger than a wavelength. So, for microwaves, the dish need only be metres in size. For radio waves, larger dishes are required.

For the dish on the ground, this is not a big problem, and dishes like the one at Tidbinbilla near Canberra are used both for transmission and reception. (There is a fun (but scientifically silly) movie called The Dish, which is about the use of the radiotelescope at Parkes--one of the world's finest astronomical instruments--for communication with Apollo XI.)

For the dish on the spacecraft, there are limits to the possible size. So the solution is to keep the wavelength short, the dish on the spacecraft smallish, and the dish on the ground big.

One the moon, in the absence of air resistance, mechanical energy of projectiles is conserved. When it returned to the same level (same gravitational PE) it would have the same kinetic energy.

On the Earth, falling small objects quickly reach a speed at which the force of drag equals their weight: their terminal speed. For a bullet, this would be several tens of metres per second, whereas a bullet is usually fired at hundreds of m/s.

Astrophysics

Resolution refers to details in space or angle. For instance, your eye has a resolution of about 1 minute of angle (about one sixtieth of a degree or 0.0003 radians). So, at 20 cms from your eye, you can resolve about 20 cm* 0.0003 radians = 0.06 mm. This is the size of a moderately large biological cell, such as an egg.

Sensitivity refers to the minimum signal required by an instrument. Larger instruments receive more light, and so (all else equal) are more sensitive. For example, under optimum, dark adapated conditions, your eye requires about 70 photons to form an image (only about 10% of these are captured by photoreceptors).

The sensitivity of telescopes is often limited by optical noise (stray light) or by electrical noise in the detectors. Electrical noise is often minimised by running the detectors at very low temperatures, where the thermal motion of electrons is reduced.

What are the the trigonometric parallax limits for space-based and ground-based methods of measurement?

Parallax refers to the different views that you see from two different positions. Try this experiment. Hold the index finger of your left hand vertical, 20 cm in front of you. Hold the index finger of your right hand vertical, 40 cm in front of you. Now close your left eye and, using just your right eye, move the two fingers sideways until they line up. Now close your right eye and open the left. The closer finger has 'jumped' to the right of the further finger. Repeat a few times. Compared to a distant background, both fingers have both jumped to the right, but the closer one jumps father. If you measure the angles through which they jump and the distance between your eyes, you can work out how far away the fingers are.

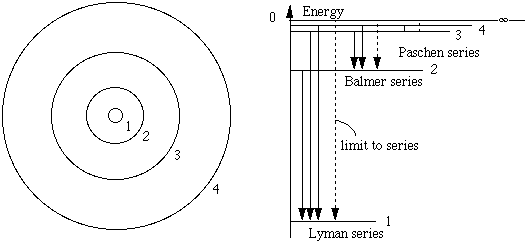
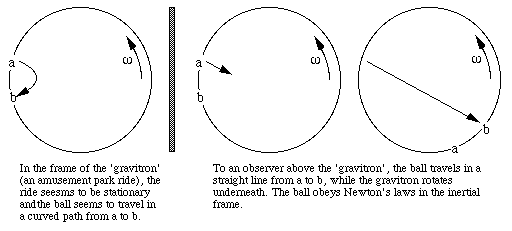
For distant objects, the distance between our viewing positions must be greater than the distance between your eyes. Fortunately for astronomers, the Earth shifts our telescopes round the sun once a year, so we can get a separation equal to the diameter of the orbit of the Earth (16 light minutes) if we wait six months, as shown in this diagram.



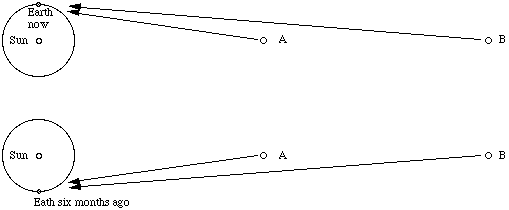
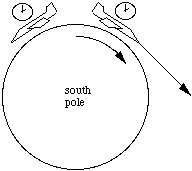
In this sketch, which is not to scale, imagine an observer looking at objects A and B, standing at the pole of the Earth with his head towards us. Now he sees object A to be to the right of B. Six months ago, he saw it to be to the left of B. Now most stars are so far away from us that we cannot observe any relative motion in this way. However, for close stars it is possible. The next sketch shows the path of light from a close object and from a very distant star.



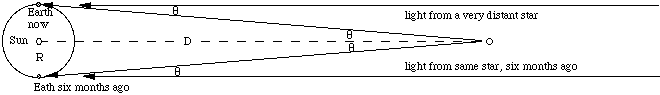
From trigonometry,

D = R/tan θ = R/θ
where we have used the small angle approximation for θ measured in radians. A parsec is defined as the distance to an object that 'moves' (with respect to the distant stars) by an angle of 1 second (1/3600 of a degree) when the Earth moves by the mean radius of its orbit. In terms of this sketch, if θ = one second, D = 1 parsec. Now all stars except the sun are more than one parsec distant, so to measure their distance by parallax, we need to be able to resolve angles of about 1 second or better. Is this possible?

One limitation to the angular resolution of telescopes is due to a wave effect called diffraction. When parallel light passes is incident on a circular lens or circular telescope with an aperture a, it cannot be focussed onto a perfect point, but rather makes a small circular smudge called the Airy disc. Around this bright circle is a dark ring, then a series of bright and dark rings: the diffraction pattern of a circular aperture. The angular diameter of the central bright ring is of the order of λ/a, where λ is the wavelength of the light (or other waves). If the angular separation between two stars is smaller than the size of this disc (as is the case for the majority of double stars), then it is very difficult to resolve them as two different stars. (In practice, this theoretical limit is not always achieved in optical telescopes because of such effects as the bending of light in the atmosphere.) So an angle of λ/a is approximately the theoretical limit to the angle that can be resolved by a telescope (or camera, or eye*).

Radio telescopes, which use long wavelengths (eg 21 cm, the wavelength of the 'hydrogen line') have to be much bigger than optical telescopes (L ~ 0.0005 mm), but in both cases, the bigger the better. Optical telescopes may have a of several metres. Individual radio telescopes may have a of 10s of m (eg the dish at Parkes is 64 m), but separate radio telescopes may be connected to provide a bigger effective aperture. The Australia Telescope links radio telescopes across the country to provide an effective aperture of thousands of km.

Space based optical telescopes have the advantage that they have no atmospheric distortion and so they can measure smaller angles than ground based ones.

* λ/a is also one of the limits to the angle you can resolve with your eye. This limit is only achieved, however, when your pupil is almost closed (aperture a less than about 2 mm), in very bright light. In dim light, when you pupil is open wider, the angular resolution is typically a bit better than one minute (1/60 degrees), and is determined by the spacing of photoreceptors in your retina (which you can now work out).

The most distant objects are reported to be about 13 billion light years away, and the universe is said to be 14 billion light years away. What stops us seeing further?

In a sense, the furthest visible thing one can see is exactly cT away, where T is the age of the universe. That is the big bang itself, which we see as the microwave background. This is microwave radiation coming almost uniformly from all directions in the sky - it is the bang, red shifted and cooled by the expansion of the universe, which makes its wavelengths longer just as it makes intergalactic distances longer. Those microwaves have taken all of the age of the universe to get here.

For localised objects, the oldest ones have to be very hot so that they are still visible with huge red shifts. And galaxies and stars didn't form for a while, so the oldest visible galaxies are a little younger than the universe.

The atom, photoelectric effect, energy levels, quanta, black body radiation,.

How does the quantisation of emitted radiation explain the black body radiation curve? Why does it have a peak?What is Wien's law?

Where exactly did E = hν come from? [snip] What exactly was the maths that Planck was trying to do that made him quantise?

The keys are black body radiation (see the preceding question) and Boltzmann's ideas in statistical mechanics. Along with others at the time, Planck was trying to explain black body radiation: the intensity, as a function of wavelength, of EMR emitted from a surface in equilibrium with its own radiation (as in the interior of a heated box: see above). Classical physics gives an expression proportional to 1/λ⁴. This is approximately what is observed for very long wavelengths. But it fails spectacularly at medium and short wavelengths, a problem known as the ultraviolet catastrophe. To write an equation that fits the data, Planck needed to put in the denominator a factor that we now write as (exp(hc/λk_BT) − 1):
B(λ,T) = (2hc²λ⁵)/(exp(hc/λk_BT) − 1).
We now recognise this as very much like the Boltzmann distribution of energies, exp(−E/k_BT), which in turn comes from the equipartition of energy among different ways of vibrating.
Proviso: actually, the one in this factor comes from the Bose-Einstein distribution, which applies to photons and other bosons, and to which Boltzmann's distribution is only an approximation. The statistics of energy distribution depends on whether particles can share quantum numbers (bosons) or not (fermions). Boltzmann's distribution was extended for bosons (photons, gluons, the W, Z, Higgs and the graviton, if it exists) by Bose and Einstein, hence the name) and for fermions (leptons and quarks) by Fermi and Dirac, hence that name. To obtain Boltzmann's distribution, consider the case of small λ, or large energy, where the 1 in the denominator is negligible.

Planck didn't like statistical mechanics and Boltzmann's work. Reluctantly, however, he found it necessary to use it. If the energy could only be emitted and absorbed radiation with energy amounts that were inversely proportional to λ (E = hν = hc/λ), then Boltzmann's equal distribution of energy among different modes would give the required short wavelength behaviour. The next step, the idea that energies of radiation were inherently quantised (a little like the way matter is quantised in atoms etc), came from Einstein's analysis of the photoelectric effect, which is our next question.

I sometimes wonder how close Boltzmann was to discovering quantum mechanics. If you think about the entropy of a single atom in a box, classical physics would give it infinite entropy. Boltzmann would have known this, and quantization of energy is only a couple of steps away... But sadly, Boltzmann was under attack from the philosophers for his insights in thermal physics and was probably not in a great position to pursue further radical implications of his ideas.

The photoelectric effect

Electron microscope

Heisenberg's Uncertainty Principle

Pauli's exclusion prinicple

The Zeeman effect, and why the Rutherford atom doesn't account for it

The spin quantum number

Let's start with some classical ideas. Angular momentum is the rotational analogue of (linear) momentum. If an everyday object is spinning, it has angular momentum. If we attach electric charge to that spinning object, the circulating charge acts like a loop of current, and produces a magnetic dipole, ie a little electromagnet.

So, when we find that an electron has angular momentum and a magnetic dipole, it is natural to talk of its spin. Natural, but somewhat misleading, because on the very small scale one must use quantum mechanics, rather than classical mechanics. Like the energy of electrons in an atom, the spin of a fundamental particle is quantised: only discrete values are allowed (+ and - 1/2 for the electron). Further, if one imagines the electron is a little ball of spinning charge and applies classical physics, one gets the wrong answer for the magnetic dipole.

So why is it a necessary concept? If we apply an external magnetic field, the energy of an electron will be increased or decreased depending on the direction of its magnetic dipole (and thus on the value of its spin). It also gives an extra quantum number. The Pauli exclusion principle forbids electrons to have the same quantum numbers so, for any energy level in the atom, there can be two electrons, with positive and negative spin. Thus spin allows twice as many electrons, which has very considerable consequences for the periodic table and chemistry!

Accelerators as probes of nuclear structure

One reason is related to the de Broglie hypothesis: that the wavelength λ of any particle is λ = h/p, where h is Planck's constant and p its momentum. So, in order to probe the nucleus (size ~ 10^-15m), we need a 'probe' particle smaller than this, which means one with large momentum. To obtain information about quarks (ie to look inside a nucleon), even higher momenta are required. Accelerators provide particles with very large momentum (travelling within a fraction of a percent of the speed of light), and the momentum is well known.

To step back in history: Rutherford was able to probe the inside of the atom by using 'probe' particles smaller than the atom. From the angles of recoil, he was able to make an important conclusion about the atom: that nearly all the mass was localised in a very small region (the nucleus).

The second reason is to do with mass-energy equivalence. If you organise a collision that, relative to the centre of mass of the colliding particles, has a kinetic energy E, it is possible to create a particle-antiparticle pair, provided that 2mc² is less than E. Smashing an accelerated particle into a target is therefore one way to study structure. One drawback is that the kinetic energy in centre of mass frame is not very high, particularly when relativistic effects are included.

A better way of studying subatomic particles is to smash a proton (mass m_p) into an antiproton. Sometimes they destroy each other, and then you get the kinetic energy of the collision, plus 2m_pc². Which may be enough to create various different particle-antiparticle pairs. Note that you usually create (or destroy) particle-antiparticle pairs, so that the total charge and spin of the things you create or destroy is zero.

Semiconductors, transistors, solar cells etc

What are n-type and p-type semiconductors?

How do diodes and transistors work?

When you take some p material and n material and put them together, you get a diode (see the schematic diagram below). If you make the p side positive and the n side negative, then holes move from the p side to the junction, while electrons move from the n side to the junction. At the junction, the electrons 'fill' the holes, thereby destroying both the free electron and the hole. So the process can continue indefinitely: the diode conducts in this direction. If you reverse the polarity, holes and electrons both move away from the junction. This leaves no charge carriers near the junction, so there is no conduction. Thus a diode conducts current in only one direction---the direction of the arrow in its circuit symbol. Diodes are useful in rectification (turning AC to DC), in logic circuits and in many other applications in electronics.


A junction transistor consists of a thin layer of one type of semiconductor sandwiched between two layers of the other type, as shown in the schematic diagrams above.

Let's look at the npn transistor. An electron can travel from the emitter (n doped) to the collector (n doped) only if it can get through the base without 'colliding' with a hole in the base (p doped). If the layer is very thin, that is possible, but the chance of an electron getting through is a sensitive function of the potential difference between base and emitter (called the bias voltage). For typical silicon transistors, if you set the base-emitter voltage at 0.2 V, there is hardly any current between collector and emitter. If you set it at 0.6 or 0.7 V, you get close to maximum collector current (the size depends upon the size and packaging of the transistor, but tens or hundreds of mA is typical). If you set the base-emitter voltage above this, you have an ex-transistor.

The pnp transistor operates similarly, except that it is the 'holes' that migrate across the thin base region, and the electrons in this region that control the flow. It is convenient to have symmetrical transistors (npn and pnp) for circuits with positive and negative supplies.

The field effect transistor or FET is simpler than the junction transistor. We show a p-gate transistor. The current through the n-doped material passes through the narrow section where it passes the 'gate' of p-doped material. The effective width of this passage can be made thinner or thicker by varying the voltage of the gate, and so removing conduction electrons from the thin passage. An advantage of FETs is that the input resistance of the device is high, which is what one usually wants when amplifying a small signal. In junction transistors, the input resistance is low. A disadvantage of FETs is that they usually can handle only small currents.

Transistors as amplifiers and logic gates

History of the invention of the transistor.

What was the impact of the invention of transistors, microchips and microprocessors on society?

More about transistors, computers

Solar cells and the photovoltaic effect

States of matter

Bose-Einstein condensates

Superconductors

It is difficult to give a qualitative/conceptual description without missing something, but here is a simplistic explanation:

Electrons in normal metals occupy a set of quantum states, up to some maximum energy (called the "Fermi energy"). The relatively free "conduction electrons" (those which come from the unpaired valence electrons of the atoms) interact strongly with the positively charged ion cores, and as an electron moves through the lattice it will cause the cores to be displaced from their equlilibrium positions. Electrons with energies near the Fermi energy are able to change their quantum state relatively easily, and thus any interaction, such as with the lattice, can result in a drastic change in the quantum states of these electrons. It happens that in superconductors the electrons near the Fermi energy become highly correlated, forming a macroscopic coherent quantum state with exotic properies. This state can be thought of as being made up of "electron pairs", but it is important to understand that these pairs are transitory things which change continuously in a dynamical way. At any instant a given electron is a member of many pairs.

Does superconductivity qualify as a new state of matter? Not according to the classification scheme proposed here. If we had a scheme that made metals a different state of matter from materials that don't share electrons, then under such a scheme superconductors might be a new state of matter. However, this is all taxonomy and semantics, and is not of great importance to physics.

What is a phonon?

The atoms of a crystalline solid form a regular lattice structure, which can be likened to an array of balls connected by springs, the springs representing forces between neighbouring atoms. Such structures can vibrate mechanically in various ways, either because of thermal motion or some external force. A sound wave travelling through a solid is an example of the latter. Quantum mechanics tells us that these vibrations can only gain or lose energy in discrete amounts and these are called 'phonons', by analogy with photons for light. Under ordinary conditions there is an enormous number of phonons or photons present and we do not see this 'graininess'. However, careful experiments confirm the picture.

What causes the lattice distortions in a superconductor?

Levitation of a magnet by a superconductor

The Meissner effect

Superconductivity and computers

Applications to magnetic fields, motors, power distributions, MRI

Nuclear physics, radioisotopes, neutrinos etc

What are some of the industrial and medical applications of radioactivity and nuclear physics?

Radioactive materials give off charged particles (electrons, antielectrons, helium nuclei) and (uncharged) neutrons, and also high energy photons (packets of light). The charged particles collide with the charged particles in ordinary matter and give off more high energy photons.

A photon has (is?) an electromagnetic field. If it has sufficient energy, it can interact with an electron and remove it from its atom. This is called ionizing radiation.

Such radiation can, in sufficient doses, kill cells because, if it strikes DNA in enough places, it disrupts the molecule and prevents reproduction.

How are isotopes used in engineering or agriculture?

An important example is in nuclear engineering: In countries such as France, the construction of power stations involves lots of nuclear engineers. One of the more important isotopes is ²³⁵U. There are also nuclear reactors used for producing diverse isotopes for medical and other uses. Some bad and good news: in various countries, engineers are involved both in the building and the dismantling of nuclear weapons.

Radioactive isotopes are used in some measurement devices. The domestic smoke detector is the most common: they sometimes use Americium. Radioactive sources are also used to measure the thickness and composition of thin films (suitably calibrated, a measure of transmitted radiation tells you how much material was present to absorbe the radiation.

How are isotopes used in agriculture?

I don't know much about this, but I'll put down these suggestions and perhaps others can add to them.

¹³C has been widely used (ie sufficiently widely that I've heard of it) in plant physiology to study the carbon cycle and photosynthesis. If you put ¹³C into a particular sugar and you find it in starch, then you know that there is a pathway from that sugar to starch etc. Often when researchers study biochemistry they choose a radioactive isotope to 'label' the biochemical in which they are interested. Then you can measure the concentration by measuring the radiation, and even better you can trace where it has come from. (Biologists would have better examples.)

As to agriculture, the link below reports the use of ¹⁵N to study root biomass and uptake, ¹³C for carbon exchange and ¹³⁷Cs for studying soil redistribution.

What is a neutrino, what is an anti-neutrino?

How is beta decay described in terms of quarks?

A neutron can decay into a proton, an electron and an antineutrino. A neutron consists of an 'up' quark and two 'down' quarks. A proton consists of two 'up' quarks and one 'down' quark. The electron and the antineutrino are both leptons. How is it that one of the quarks appears to have changed from a 'down' quark to an 'up' quark?

A neutron consists of "up" and "down" quarks:    n = (udd).
Similarly, a proton is                         p = (uud)
The electric charge of the u quark is 2/3 (in units of the elementary charge) and the electric charge of the d quark is -1/3. As a result the proton has charge 1 and the neutron is neutral. The neutron decay goes via the following mechanism:

The d quark decays into u quark and a virtual W^- boson. As a result of the decay, the neutron is converted to proton. The virtual W^- boson lives for 10^-26 seconds and than decays to electron and antineutrino. Blink and you miss it!

Medical Physics

Magnetic focusing

Motors and Generators

Sorry, I cannot see any important direct relevance. The equation refers to infinite wires, ie wires whose separation is much smaller than their lengths. So the forces that make a motor work don't relate to this equation. The forces between say elements of wire in a stator coil and a rotor coil that are parallel are calculated using the Biot-Savart law rather than this equation. The only places where lengths of wires in motors are much closer than their length are between adjacent wires in the coil winding. And yes it's true that there would be a force tending to push the wires closer together, but as the wires (or their lacquer) are touching already, this doesn't have much effect.

Indirectly, one could say that the equation that you quote defines the ampere. So many electrical measurements on motors depend indirectly on this equation.

Internal forces in a rigid object have no direct effect on its motion. If the force were large enough to change the geometry of the coil, then there could be an effect, but this would be very small for any normal coil.

The wires must be very close together for the force to be important. In power distribution, the wires are usually separated by distances that make the force tiny. In transformers, wires are wound closely together and they exert larger forces on each other. I expect that transformers are tightly wound so that they don't rattle.

Transformers

Transformers in electricity supply and the home

Why does one need to have transformers in the transfer of electrical energy from a power station to its point of use? Much of the energy generated by a power station is lost in ohmic losses in the distribution network. The power lost in a wire of resistance R is R*i². To make R small requires thicker wire, which is expensive. So i is made smaller. To deliver the same power P = Vi, V must be made bigger. So, near the power station, a 'step-up' transformer steps the voltage up to a high value (e.g. 110 kV). At local substations and surburban transformers, this is successively stepped down to 240 V.

Discuss why some electrical appliances in the home that are connected to the mains domestic power supply use a transformer Many appliances require low voltage for solid state electronics (transistor circuits typically require 5 - 20 V DC) or for safety. (eg a low voltage electric toothbrush is safer than a 240 one).

Some appliances require high voltage (neon lights, the cathode ray tubes in TVs and computer monitors).

The usual approach is to use a transformer to get the voltage to the appropriate value, and then (if required) a rectifier, capacitors and regulator to convert AC to DC. (There are also DC voltage converters that 'chop' the AC on and off, allowing capacitors to charge to the desired voltage. These do not need transformers.)

How is heating caused by eddy currents in transformers overcome?

Laminating the core reduces the heat loss. The core is made out of strips of metal, separated by lacquer or other insulator, so the area of the eddy currents is reduced. Consequently, the flux and therefore the Faraday emf are smaller, so smaller eddy currents flow. You may notice transformers buzzing at 100 Hz (near G at the bottom of the bass clef) as the laminations all become electromagnets 100 times per second.

Eddy current heating is never completely overcome, and transformers are usually at least a little warm. (In Sydney, cockroaches like to live on or near transformers because they are warm.)

How does the principle of induction apply to cooktops in electric ranges?

Some electric ranges have a coil of wire instead of a hot plate. This carries AC and produces a strongly varying magnetic field. When a conducting saucepan is placed upon it, eddy currents are induced in the metal in the base of the saucepan. These produce heat via ohmic losses (and hysteresis in magnetisation).

One could consider the coil in the cooktop as a primary and the metal in the saucepan as the secondary of a transformer.

As the heat is produced directly in the saucepan itself, less heat is wasted in the cooktop or the air.

There is a further subtlety. Let's compare a pot made from aluminium , which is non-magnetic but has low resistivity, with one made from iron, which is magnetic but has rather higher resistivity. If the two were placed in the same magnetic field varying in the same way, the Faraday emf would be the same. The ohmic power loss in the two would be given by V²/R, where V is the Faraday emf and R the resistance. R would be lower in the Al, so the ohmic power loss would be less.

However, there are three complications to this argument. First, the magnetic field in the Al pan will be less, because it is non-magnetic. This makes it a less effective transformer, just as an air-cored transformer is less effective than an iron cored one in low frequency applications. (Technically, we say that the magnetic permeability Al is much lower than that of Fe. The ratio is a few thousand times, so the effect is large.) Second, a higher secondary current actually decreases the magnetic field, because it provides a back emf in the primary. And thirdly, ohmic losses are not the only losses. Energy is also lost in magnetising and demagnetising the iron each time (technically hysteresis losses). So for these three reasons, the heating in the iron pot will be greater than in the Al pot.

How are eddy currents used in switching devices?

Proximity switches often involve eddy currents. These switches have the advantage that they have no (internal) moving parts or electrical contacts. This is an advantage because springs and bearings can wear out and because electrical contacts can become corroded. Proximity switches are activated by the proximity of a conductor, such as a human finger. You will have noticed them in the control panels of lifts, for example. If the proximity of your finger throws the switch but the proximity of a non-conductor does not, the switch may work on eddy currents (although it could also work by capacitance). Proximity switches of one type work like this:

The switch contains an oscillator using a resonant circuit operating at radio frequencies. The coil sets up a high frequency magnetic field. When a conductor enters this field, eddy currents flow in the conductor. This can have two effects. First, it changes the impedance of the coil, and so changes the frequency* of the resonant circuit. Also, because of ohmic losses in the conductor, energy is lost from the resonant circuit into the conductor. This may be large enough to stop* the oscillations. One or other of these changes then activates the switching circuitry. The actual switching of a high current circuit might achieved by a solid state device (MOSFETs, TRIACs etc) but for high current applications a relay is often used.

* You can think of the coil and the conductor as the primary and secondary of a transformer. The resistive load of the secondary is 'reflected' into the primary circuit, changing its properties. Note that both the frequency and the Q value of a resonant circuit depend on the resistance of the coil.

Eddy current braking

The deceleration due to friction braking is limited (i) by how much normal force you can apply to the brake pad or brake shoe (often not a problem with servo-assisted hydraulic cylinders application), (ii) by the mechanics and size of the pad: it may shear or break up if the forces are too great and (iii) by what to do with the heat generated. The last is quite important: think of how much kinetic energy a train has at speed: if you turn that into heat in a series of small brake pads they may melt, vaporise, weld to the wheels etc.

The deceleration due to magnetic braking is limited by (i) the strength and size of the magnetic fields available, (ii)

Oscilloscope

The timebase affects horizontal motion of the beam. (The voltage inputs affect vertical motion of the beam when in 'timebase' mode, but in X-Y mode, the two different input voltages deflect in the X and Y directions.)

The electron beam in a CRO can be deflected left-and-right or up-and-down by electric fields. These are produced between two metal plates which have a potential difference supplied by the horizontal and vertical amplifiers.

In the timebase mode, a voltage which increases linearly with time is generated and input to the horizontal amplifier: this sweeps the beam smoothly from left to right. (It then starts again from the left, at a time determined by the trigger, so the timebase waveform is actually a sawtooth. You never see this waveform on the screen, however: it's used to drive the beam.)

Without any voltage input, the timebase thus causes the oscilloscope to "draw" a horizontal line across the screen. The speed of 'drawing' the graph depends on the timebase settings, sometimes called the SWEEP SPEED.

This control knob (usually towards the right) sets the time axis so that that one division represents the time interval indicated around the dial from seconds (fully counterclockwise) down to microseconds (fully clockwise). If you set this knob fully counterclockwise, the beam will sweep so slowly across the screen that you can see it cross. Fully clockwise and the sweep will look like a continuous line, because your eyes are not fast enough to see the motion.

Simple Harmonic Motion under gravity

We attach a mass m below a vertical spring with constant k (fig b). At equilibrium, the spring is extended a distance x, where
kx = mg.



Let us now measure position with respect to this equilibrium position, using the new variable y. For instance, we might apply a steady force F vertically and achieve a new equilibrium at position y (fig c) where

F + k(x - y) = mg.
Substituting for x gives us
F + mg - ky = mg,     or
F = ky.
Put in words: the weight of the mass and the force due to the equilibrium displacement of the mass cancel out. Displacement from this equilibrium position requires a force ky, and so the potential energy at position y (with respect to y = 0) is
U_total    =    integral of ky dy  =   (1/2)ky²
This potential energy could be said to include a compent due to the spring and another due to gravity. The choice of a reference for potential energy is arbitrary. Let's take it instead with respect to the unstretched position, and signify this difference with a prime.
U'_total  =   U'_grav + U'_spring   =   (1/2)k(x - y)² + mgy

=   (1/2)kx2 - kxy + (1/2)ky2 + mgy

=   (1/2)kx² + (1/2)ky²

where the first term is a constant (the spring energy at equilibrium) and the second term is the energy required to displace it from that position.

So, from both the Newtonian and Hamiltonian points of view, the mass on the spring in the gravitational field behaves exactly as it would in the absence of gravity, except for the altered equilibrium position.

Drift velocity

Miscelleaneous questions in history and social studies

2001 HSC Paper on Physics

Both answers are correct. B is true: both generators produce AC. C is also true: Generator 1 produces DC and Generator 2 produces AC.

This comes about because generator 1 produces both AC and DC at the same time (ie a current that has both an AC and a DC component.

The splits in the ring in generator 1 are in such a position that the circuit is reversed at two orientations when the flux is changing at a reasonably high rate, so it goes quickly from an emf in one direction to an equal emf in the other. The current produced would in the shape of sin(ωt) for half a cycle then −sin(ωt) for the other half cycle, but it doesn't change at π/4 or 3π/4 radians, so the output has some DC component, and a larger AC component. Exactly where it changes depends on how you estimate the angle in the drawing. The current will not be discontinuous because of the inductance of the coil, and there will be some spikes and sparking.

So the examiners should have marked both B and C correct.

Q3. 'Resistance' should be 'Resistivity', or else there should be some words between 'resistance of' and 'mercury'. The question as asked is dimensionally incorrect. This error is unlikely to have confused anyone.

Q28 The quantity should really be the 'specific acoustic impedance' or 'wave impedance', rather than the 'acoustic impedance', but this is unlikely to have confused anyone.

2003 HSC Paper on Physics

Glossary and skill list

Hints on doing tests

Other useful links