Relativity in brief... or in detail..

Anwers to the Quiz: how well have you understood the Einstein Light presentation?

The questions appear with their answers below.

Question 1

The chemist and the accountant. My chemistry text tells me that 12.000 g of carbon 12 is called a mole and has 6.023 x 1023 atoms. Each atom has six protons, six neutrons and six electrons. When I look up the mass of protons, neutrons and electrons, I find that the whole is less than the sum of the parts. Carbon 12 weighs less than the combined weights of its protons, neutrons and electrons. What is the explanation?
    Answer: Cabon is a stable element: its atoms do not spontaneously divide. This is because attractive forces hold its nucleus together. (See Why there would be no chemistry without relativity for details.)

    Consequently, it takes work to 'pull a carbon nucleus apart' into its constituent neutrons and protons. This work W increases the mass by an amount Δm where W = Δmc2.

Question 2

A bus turns a corner to the right and a standing passenger, who is not holding on to the grab rail, falls over. Explain this briefly in terms of Galilean or Newtonian physics.
    Answer: Okay, imagine the situation. You are standing, the bus turns sharply right. You fall to the left. The startled neighbour into whose lap you have fallen demands an explanation.
      "It was centrifugal force made me do it" you explain.
      "Garbage" she says. "Centrifugal forces don't exist. They are called fictitious forces and your explanation is a fictitious explanation."

      The confrontation escalates and we discover that a policeman has seen the bus turn. "Ah", she says "an observer in an inertial frame of reference: he'll be able to explain it".

      "Easy" says the policeman , who has just read Inertial frames and why the laws are the same in the train and on the platform, "There was no centrifugal force. You kept travelling in a straight line while the bus, including your squashed interlocutor, turned right. She put her lap immediately under your trajectory.

    The gradually reflating victim accepts this explanation and your apologies, while you consider accusing the bus driver of facilitating an improper assault.
      "Meanwhile", says the policeman, "regard this as a caution. It's not enough just to obey Newton's laws - even hardened criminals do that. You should hold onto the grab bar as well."
    Or, more prosaically: the cornering bus is not an inertial frame: Newton's laws do not apply when referred to that frame. A stationary observer outside the bus is in a frame that is nearly inertial. This observer sees the passenger continue forward, initially in a straight line, while the bus accelerates to the right. Within the frame of the bus, he appears to travel to the left side of the bus. The friction between his shoes and the floor pull his feet to the right, so his feet are no longer below his centre of mass.

Question 3

Imagine that you are travelling in a spaceship at 99% of the speed of light. You look at your watch. Do you see anything strange? Explain your answer.
    Answer: No. An observer outside would see that the watch ticked slowly, and would have changed its shape, being shorter in the direction of travel. However, your body clock - your internal sense of time - is determined by electric forces, as is the watch. Both are slowed by the same factor, so you see no slowing of the watch. Similarly, in your frame, everthing has its proper length. (See Relativistic time dilation, simultaneity and length contraction for details.)

Question 4

If the laws of physics are the same for two observers in uniform relative motion, why do they get different answers when they calculate physical quantities? Explain briefly, perhaps with an example.
    Answer: Because of the relative motion. For instance, one observer might see stationary charges, and hence no magnetic force, while another could see moving charges, and hence a non-zero magnetic force, as in our example in Module 2.

Question 5

When Jasper and Zoe board space ships in Energy in Newtonian mechanics and in relativity, why does Jasper's ship change colour and not Zoe's? (Hint: in deep space, where does the light come from?)
    Answer: Note that both space ships are seen from the side so, when light left them, they were travelling at right angles to the observer. So there is no Doppler effect of the ordinary kind. In deep space, there is only starlight: it is typically as bright as on Earth on a moonless night. Not bright enough to see colour. So the space ships must be providing their own light. Viewed from outside, the relativistic ship has a time dilation and, as v gets larger, the oscillations giving rise to the light are slower, so the frequency is lower, the wavelength is longer and the colour is redder.

    This question is arguably a trick question, because of the severe simplifications that have been made in the animations, as discussed in the Caveats.

Question 6

An astronaut set out in a spaceship from Earth to travel to a distant part of our galaxy. The spaceship travelled at a constant speed of 0.8 c. When the spaceship passed a certain star, the on-board clock showed the astronaut that the journey had taken 10 years. To save you punching a calculator, at the speed 0.8 c, the relativistic factor g = (1-v2/c2)-.5 = 1.7 and its reciprocal is 0.6.

An identical clock remained on Earth. What time had elapsed on the Earth clock, when the astronaut's spaceship passes the star?

    (A) 4 years
    (B) 6 years
    (C) 10 years
    (D) 17 years
    (E) none of the above
    (F) any of the above
This only looks like a multiple choice question: what is of real importance is that you justify the answer. Award yourself points only for the correct explanation. And beware: this question is not as trivial as it looks.

    Answer: Beware the word "when". In normal use, this word implies simultaneity. In relativity, if you refer to two distant events, simultaneity can be in the watch of the beholder. Here, no frame was specified, so the answer is (F).

Question 7

A scientist measures the current flowing in two long parallel wires. The flow is in the same direction in each, as in our demonstration. Knowing the density of conduction electrons and the cross section of the wire, she works out the average speed of the electrons in the wire. Let's assume it is the same for all conduction electrons, and zero for all others. The speed v of the conduction electrons is very much smaller than c, as is usually the case. Also, let us neglect the voltage difference along the wire (the resistance of the wire is negligible).

She now decides to observe the two wires from a high speed vehicle travelling parallel to the wires, in the direction of the electron travel, at the same speed. Will the force between the two wires be larger, smaller, about the same, or zero? Again, marks for the explanation of the correct answer, not just for the answer. (If the electrons move at speeds that are not << c, then this question becomes much more complicated.)

    Answer: The high speed observer sees the conduction electrons at rest, but the wires (including all of the positively charged ions that consitute atoms minus conduction electrons) travelling in the opposite direction, but at the same speed as she previously recorded for the electrons. So she will see an attraction between them (which is just as well, if she has to explain an acceleration or a bending of the wires). If we neglect relativistic effects, the situation is almost symmetric and the force is about the same. (Two Newtonian observers get the same value for the force.)

Question 8

A long pendulum is set swinging in a plane, somewhere on the surface of the Earth. An observer notices that the plane of the oscillation of the pendulum appears to rotate anticlockwise such that it appears to be again swinging in the same plane about 12 hours later. Is it raining?

    Answer:At the pole, the pendulum swings in a plane that, from an inertial frame, does not precess. So, to an observer on the (accelerating frame of the) Earth, it appears to precess 180 degrees in about 12 hours. At the equator, from symmetry, there is no precession, so the precession time is infinite. At all other latitudes, the precession time lies between these two extremes. See the Foucault pendulum.

    So this pendulum swings at the South Pole, where it never rains.

Question 9

A train moves at constant, relativistic speed. When the train passes a pole by the track, a clock on board records the time reading. There are two poles. Each has a similar clock, which records when the front of the train passes it. The clocks on the poles have been carefully synchronised.

Call Ttrain the interval measured between the readings by the clock on the train, and Ttrack the interval between the readings of the pole clocks.

Consider the following statements:

    i) According to relativity, an observer by the track sees the clock on the train running slow, so he predicts Ttrain < Ttrack.
    ii) According to relativity, an observer on the train sees the clocks on the poles running slow, so she predicts Ttrack < Ttrain.
    iii) The two observers can send each other their (already recorded) measurements, at their convenience. They cannot both be correct, so there must be a paradox.
Is this a paradox? If not, why not? (And if so, what shall we do with all those textbooks about relativity?)

Question 10

Zoe has entered her car for a new event: the Bathurst Time Trial. Her car (a '61 Holden fitted with tail fins to increase speed) is believed to be so fast that a special timing system has been devised. It works like this: at the start, a laser in the car sends a beam laterally towards an array of mirrors set up at the half-way point of the course, as shown in the two diagrams below (not to scale). The course has length L and the mirrors are at different distances wn from the track, as shown in the sketch. (The mirrors are also at slightly different heights and the beam is spread in the vertical direction so that it can hit all of them, but this detail is unimportant for the following question.)

At the finish line, a reflected beam is received by one of a vertical array of detectors fixed to the side of the car. From the height of the detector that received it, one can tell which mirror reflected the beam. The further the reflector is far from the track, then the further light has travelled during the time trial, so the slower the car. The aim in this race is to have a low value of wn.

diagram of the race timing, beginning    diagram of the race timing, beginning

i) The judges of the race determine that the pulse received by the car at the finish line was reflected by the nth mirror, at a distance wn from the track. From this observation, the judges then calculate their value of the time tjudge taken by Zoe for the event. Derive an expression for tjudge in terms of L, wn and c, the speed of light.

    Answer: For the judges, using Pythagoras' theorem, the light beam travels
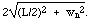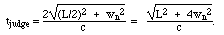
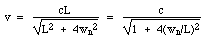      equation
    It travels at c, so the time taken by the car is
      equation
ii) Using your result from (i), give an expression for the speed v that the judges will record for the event. Express your answer in terms of c and the ratio wn/L.

    Answer: For the judges, v = L/tjudge, so
iii) Rearrange your answer to (ii) to express L/wn in terms of c and v.

    Answer:
      equation
iii) Zoe also observes that the light has been reflected from the nth reflector. From this observation alone, and without using the Lorentz transformation equations, give an expression for the time tZoe that Zoe will calculate as the time she took to complete the distance L. Your expression should not involve v.

Explain in one or two sentences how you derived your answer.

    Answer:
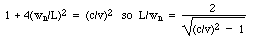      equation
iv) Zoe and the judge determine different times: tjudge does not equal tZoe. Nevertheless, from independent measurements such as the Doppler shift in light, they both agree on the speed v.

Describe how Zoe (who understands relativity as well as motor mechanics) would explain the difference between the two results for tjudgeand tZoe.

    Answer: From Jane's point of view, the light beam travels at right angles to the car: it goes out to the right, strikes the reflector when the car is at the halfway point, and arrives at the detector at the finish. She is an inertial observer, so, according to the principle of special relativity, light travels at c with respect to her. Hence the time taken is
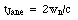
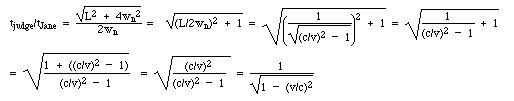
      equation
v) Describe how the judges (who also understand relativity) would explain the difference between the two results for tjudge and tZoe.

    Answer: The judges would conclude that Jane's clock is running slow. It has suffered a relativistic time factor due to its speed v, relative to them. (Note that the judges can measure the proper length L.)
vi) From your results above, give an expression for the ratio tjudge/tZoe. Using your expression for part iii to simplify your answer, express it in terms of the ratio (v/c) and comment briefly.

    Answer:
      equation
vii) The laser (a gas laser) has a tube mirror at either end, with a standing light wave between the mirrors, as shown in the inset at bottom right. At what angle to the direction of the car should the laser point so that the beam will strike one of the reflectors and return to be picked up by the detector in the car at the finish line? Your answer should have a sentence or two of explanation. It should include a sketch of the situation in the frame of the judges.

    Answer: As described in (iv), from Jane's point of view, the laser beam travels at right angles to the car: so it should be pointed in this direction. Viewed from the judges' frame of reference, both the light inside and outside the laser travel at the same angle to the car, but pointing the laser at right angles is still correct, as illustrated in the sketch.

sketch of laser moving in transverse direction

Question 11 (A symmetrical 'twin paradox').

Two space travellers are initially at rest, each at a large distance L from a central clock, but in opposite directions. On their twentieth birthdays, each determines that the central clock records the same time. The light has taken the same time to reach each traveller, they are both at rest in the same inertial frame so, in this frame, they are equally old. Complete symmetry.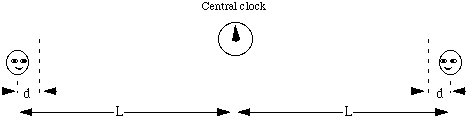
    diagram of symmetric twin paradox
They set off to meet at the central clock. Over a distance d, which is negligible in comparison with L, they accelerate to speeds comparable with c. Everything is symmetrical.

During the unaccelerated flight, they are travelling at relativistic speeds with respect to each other. Consequently, during that part of the trip, each traveller sees that other's clock is running slow, and each sees that the other is ageing more slowly. (And remember that d<

Is this a paradox that destroys relativity? If not, why not?

    Answer: The solution to this apparent paradox is in the last few paragraphs of The Twin Paradox.

    In a frame of reference that is accelerating, you must apply General Relativity. Special Relativity on its own applies only to inertial frames of reference. So Special Relativity alone does not give the right answer in this case.

    In this case, the twins accelerate from rest and, while they are accelerating, they know that they are not in an inertial frame: they are pushed against their seats by mysterious forces, etc. Yes, one could make d very small, but then the acceleration would have to be greater to achieve relativistic speeds.

    During the accelerating phase, general relativity must be applied. As we explain in the Twin Paradox, under general relativity, an accelerating frame of reference is locally indistinguishable from a gravitational field. So each twin could see the other as being a height 2L above himself in a uniform gravitational field. At high gravitational potentials, general relativity shows that clocks run more quickly, and the effect is proportional to the potential and so proportional to 2L. (Further, if you make d smaller, then the acceleration to reach the same speed is larger, so the effect is more intense but shorter.) There is also the usual correction for their relative motion (the effect we should get from applying special relativity alone) but, during a sufficiently rapid and distant acceleration, the "special relativity" term (the one that makes clocks run slowly) can be made negligible in comparison with the "general relativity" term (the one that makes clocks run quickly). Both terms are completely symmetrical.

    Consequently, each twin sees that the other ages rapidly during the acceleration (and the larger L is, the more rapidly the other is seen to age). Then, during the unaccelerated part of the flight, each sees the other age more slowly (the term due to relative velocity, what we could call the "special relativity term", dominates because there is no general relativity term). These two effects cancel out so, when they flash past each other at the central clock, they are the same age.

    Each agrees that they first fire their rockets simultaneously. Each observed that the other's clock ran more quickly than theirs during the distant acceleration, and then each observed that the other's clock ran more slowly than their own during the unaccelerated phase. Complete symmetry: when they passed each other, they were the same age.

    Alternatively, if you draw a space time diagram, and include birthday greetings as we did in Twin Paradox, then you will also see that, in all frames, each twin receives the same number of birthday greetings from the other.

    It is a neat question, and my correspondent really thought that he'd found an inconsistency in relativity. I also thank this correspondent, who had obviously thought about relativity and who took the time to express the question as elegantly and clearly as possible. (This is not as common as one might hope. See Einstein was wrong.)

    However, to all those who feel frustration that Einstein always 'gets away with it' -- that relativity always gives correct and logically consistent answers -- there is this consolation: Despite his important contribution to Quantum Mechanics, Einstein never really accepted the fundamentally stochastic nature of the universe at the small scale. So he posed a series of puzzles to Neils Bohr and others, in which he attempted to show inconsistencies in Quantum Mechanics. In all cases, Einstein's interlocuters were able to show that Quantum Mechanics was right and Einstein was wrong. With co-authors Podalsky and Rosen, Einstein wrote a paper proposing an explicit experiment (the EPR experiment) to test Quantum Mechanics in this way. He did not live to see any of the (approximately equivalent) experiments performed (by Alain Aspect's team in 1982 and by many others since). However, the results are unanimous, and Quantum Mechanics is under no threat -- or at least not from that direction. A nice summary, with links to the original papers, is given by David R. Schneider.

Home | Summary | Quiz | Credits
School of Physics - The University of New South Wales
Sydney, Australia 2052 © School of Physics UNSW 2052 Disclaimer
feedback